Figuring Out Figurate Numbers

Scenario

The figure below shows the visual pattern for the first few triangular, square, pentagonal, and hexagonal numbers. These numbers are called figurate numbers . The number of dots in an individual polygonal shape represents a particular figurate number for each pattern. For example, the first five triangular numbers are 1, 3, 6, 10, 15. The first four square numbers are 1, 4, 9, 16, and the first three pentagonal numbers are 1, 5, 12.

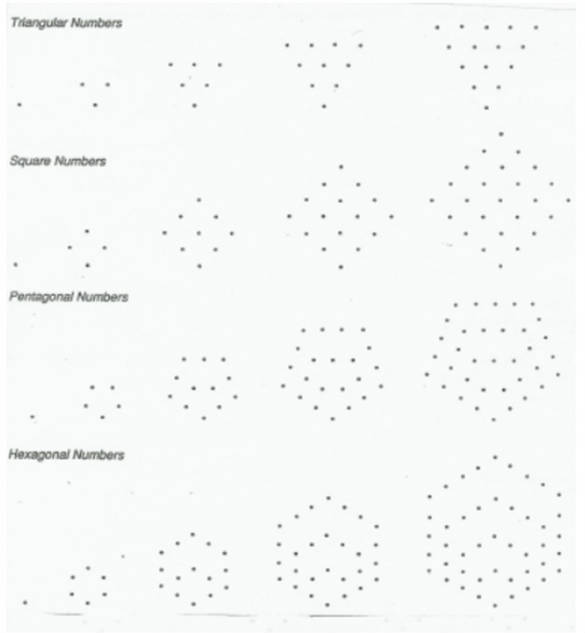
The n th square number is n² . Each n th triangular number is the sum of the first n positive integers: 1, 1 + 2 = 3, 1 + 2 + 3 = 6, 1 + 2 + 3 + 4 = 10…. The recursive process of producing this number can be visualized as adding a row of n dots to the top of the previous triangular number to obtain the next one in the pattern. Your students may or may not already know a quick way to find the n th triangular number.

What are the n th pentagonal and hexagonal numbers?
Construct the pattern for the first five heptagonal and octagonal numbers, respectively. What are their n th numbers?

Extension: Can you find a way to represent the n th p -agonal number, where p is the number of sides of a regular polygon?