By Isil Isler, Ana Stephens, and Hannah Kang, posted January 18, 2016 –

The notion that elementary school students can and should engage in algebraic thinking is being increasingly accepted and advocated. We have found that the four algebraic thinking practices of generalizing, representing, justifying, and reasoning with mathematical relationships (Blanton et al. 2011; Kaput 2008) provide rich experiences to engage elementary school students in early algebra. In this post, we focus in particular on the practice of justifying. Asking students to justify their responses can help us assess their understanding as well as introduce them to an important part of what it means to do mathematics. The earlier students become accustomed to justifying their responses, the sooner they get into the habit of thinking that mathematics should make sense. Constructing viable arguments and critiquing the reasoning of others is one of the Common Core’s Standards for Mathematical Practice (SMP 3) that is closely related to the algebraic thinking practice of justifying.

As an example of how students can engage in the practice of justifying, consider the following activity that we implemented with third-grade students.

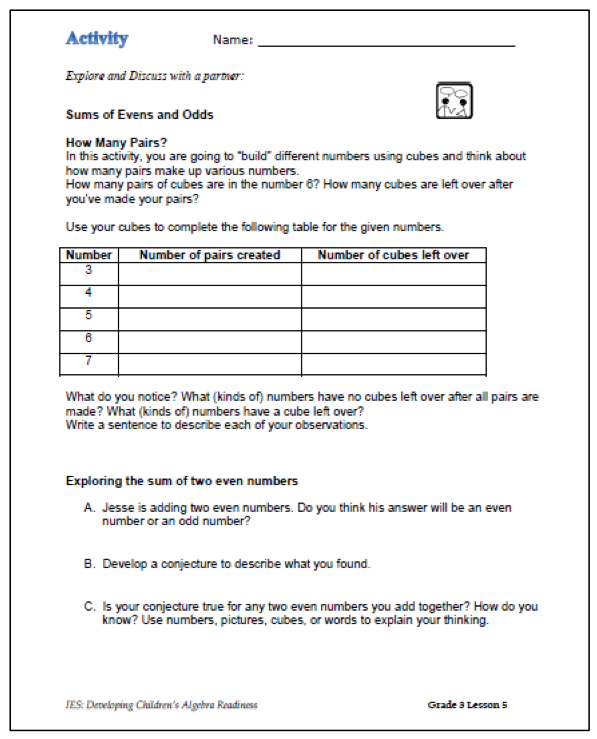
Our goals with this activity were to have students explore even and odd numbers, develop conjectures based on the sums of even and odd numbers, and justify their conjectures using numbers, pictures, cubes, or words. Problems involving the exploration of even and odd numbers can provide a good context to start engaging students in justification and proof. Having students work with manipulatives and record the patterns they notice in tables can support their development of definitions of even and odd numbers, which can in turn help students construct generalizations about these numbers. In the elementary grades, students usually define even numbers as “numbers without leftovers when broken into pairs,” and odd numbers as “numbers with one leftover when broken into pairs” (see the related Common Core Standard 2.OA.3).

One particular item that we have used to assess students’ abilities to justify in grades 3–5 is the sum of three odd numbers task (adapted from Knuth, Choppin, and Bieda 2009):

Brian knows that anytime you add three odd numbers, you will always get an odd number. Explain why this is always true.

Before sharing sample responses from our students, we invite you to think about expected student responses for this task as well as what kind of response you would consider acceptable and why. Carpenter, Franke, and Levi’s (2003) justification framework provides us with a way to think about levels of sophistication in students’ justifications. They propose that students’ justifications tend to fall into three broad categories:

1. Appeal to authority

As you may have already observed in your class, many students, when asked to justify their responses, say, “I know because it is in the book” or “My teacher (or parents) told me.” This type of response suggests that the student accepts a given argument without question and that he or she believes it is true because it has been said so; these responses are categorized as “appeal to authority.”

2. Justification by example

This category is characterized by students’ reliance on examples to justify that a conjecture is true for all numbers. Students usually try a few examples to test the conjecture, and on the basis of what they find, they claim that it works for all numbers. For instance, when asked why the sum of two even numbers is even, a student might say, “The sum of two even numbers is always an even number because 4 + 2 = 6 and 6 is even.” Although examples can be useful in helping students “test” whether the conjecture is true for those cases, they cannot prove that it is true for all numbers. (Examples cannot prove that a conjecture is true for all numbers; however, a counterexample is enough to disprove that a conjecture is false.) Students might use examples because they don’t have the necessary “tools” to go beyond examples. Therefore, we should help students see the limitations of examples, and produce general arguments.

3. Generalizable arguments

In this last justification level, proposed by Carpenter et al. (2003), students provide general arguments, which are usually based on the definitions of even and odd numbers or built on already-justified conjectures about sums of even and odd numbers. An example is “The sum of two even numbers is always an even number because even numbers can be divided into pairs with no leftovers, and if you add two numbers with no leftovers, the sum does not have any leftovers.” Students may use manipulatives or pictures to explore and justify the conjectures.

Your Turn 

We encourage you to consider the ideas above and try them with students if you are able. In the next blog post, we will look at some examples of students exploring the idea of adding odd numbers. We want to hear from you! Post your comments below or share your thoughts on Twitter @TCM_at_NCTM using #TCMtalk.

References

Carpenter, Thomas P., Megan Loef Franke, and Linda Levi. 2003. Thinking Mathematically: Integrating Arithmetic and Algebra in the Elementary School. Portsmouth, NH: Heinemann.

Common Core State Standards Initiative (CCSSI). 2010. Common Core State Standards for Mathematics (CCSSM). Washington, DC: National Governors Association Center for Best Practices and the Council of Chief State School Officers. http://www.corestandards.org/wp-content/uploads/Math_Standards.pdf

Kaput, James J. 2008. “What Is Algebra? What Is Algebraic Reasoning?” In Algebra in the Early Grades, edited by David W. Carraher and Maria L. Blanton, pp. 5–17. New York: Lawrence Erlbaum Associates.

Knuth, Eric J., Jeffrey M. Choppin, and Kristen N. Bieda. “Middle School Students’ Production of Mathematical Justifications.” 2009. In Teaching and Learning Proof across the Grades: A K–16 Perspective, edited by Despina A. Stylianou, Maria L. Blanton, and Eric J. Knuth, pp. 153-170. Studies in Mathematical Thinking and Learning Series. New York: Routledge.

National Council of Teachers of Mathematics (NCTM). 2011. Developing Essential Understanding of Algebraic Thinking for Teaching Mathematics in Grades 3–5, edited by Maria Blanton, Linda Levi, Terry Crites, and Barbara Dougherty. Essential Understanding Series. Reston, VA: NCTM.

Russell, Susan Jo, Deborah Schifter, and Virginia Bastable. 2011. Connecting Arithmetic to Algebra: Strategies for Building Algebraic Thinking in the Elementary Grades. Portsmouth, NH: Heinemann.

Schifter, Deborah. 2009. “Representation-Based Proof in the Elementary Grades.” In Teaching and Learning Proof across the Grades: A K-16 Perspective, edited by Despina A. Stylianou, Maria L. Blanton, and Eric J. Knuth, pp. 87–101. New York: Routledge.

Dr. Isil Isler, [email protected], is a recent graduate of the University of Wisconsin–Madison. She is interested in algebraic thinking, and reasoning and proof in the elementary and middle grades. Dr. Ana Stephens, [email protected], is an associate researcher at the Wisconsin Center for Education Research at the University of Wisconsin–Madison. She is interested in the development of students’ and teachers’ algebraic reasoning and helping teachers focus on students’ mathematical thinking. Hannah Kang, [email protected], is a Master’s of Science candidate at the University of Wisconsin-Madison. She is interested in equity and diversity issues within mathematics education, as well as students’ algebraic thinking. 

The research reported here was supported in part by the National Science Foundation (NSF) under DRK-12 Award No. 1219605/06. Any opinions, findings, and conclusions or recommendations expressed in this blog are those of the authors and do not necessarily reflect the views of NSF.